Solving Multi Step Equations Answers
Whether yous're new to solving multi-step equations or merely studying before that large chapter exam, Albert has yous covered!
This blog mail service will guide you through defining multi-step equations, examples of multi-step equations, and how to solve multi-step equations (including problems with fractions and words). Let's go!
Return to the Tabular array of Contents
What is a multi-pace equation?
Call back, an equation is a mathematical sentence that uses an equal sign, = , to show that ii expressions are equal.
We began our study of solving equations with ane-step equations, and so we moved on to 2-step equations. (Check out those links if you need a quick refresher!)
At present nosotros are moving to multi-step equations. A multi-step equation is an equation that takes two or more steps to solve. These problems tin can accept a mix of addition, subtraction, multiplication, or sectionalisation. Nosotros likewise might have to combine similar terms or utilize the distributive holding to properly solve our equations.
And so become your mathematical toolbox out! Yous never know what you lot might run into in a multi-step equation!
Examples of multi-pace equations
Multi-footstep equations are a broad-ranging category of equations. Some can be very simple, while others get more complex. Never fright! Nosotros're going to show you many examples of multi-step equations and how to solve these important aspects of Algebra 1.
Here are some examples of multi-step equations:
5x + x = 3x + 12
8y - 3 - 2y = 5
4(3m - 2) = 16
5x - 10 + 5 = 20 - 5x
Return to the Table of Contents
How to solve multi-step equations
Remember, an equation is solved when we have isolated the variable and found a value that makes the equation truthful. In order to solve equations, we use inverse operations to help u.s. isolate the variable.
\text{Addition} \leftrightarrow \text{Subtraction}
\text{Multiplication} \leftrightarrow \text{Partition}
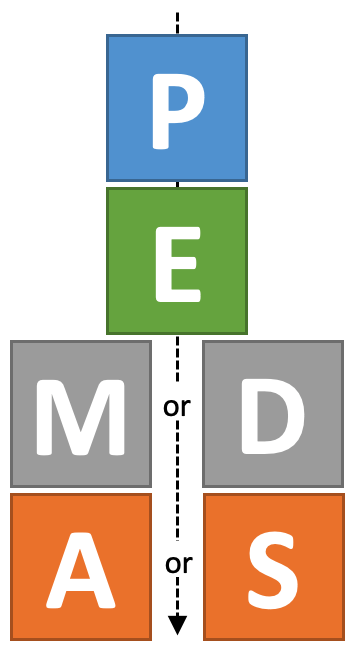
Order of Operations
Another mathematical concept that will aid when solving multi-stride equations is the Order of Operations. To use the social club of operations, nosotros must first do whatsoever operations inside grouping symbols (parentheses, brackets, etc), then exponents, then multiplication or partition (whatever comes first, left to right), then finally addition or subtraction (whatever comes first, left to correct). You can remember this by the acronym, PEMDAS.
Additionally, nosotros may take to combine similar terms on either side of the equation to assist solve these equations. Eventually, you will create a one- or 2-step equation that you will be able to solve similarly to previous problems!
Hither is an example of a multi-step equation with variables on both sides:
Solve for x in the following equation:
| 8x - 10 = 4x + 2 | Original equation |
Since at that place are variables on both sides, we must eliminate the variable from one side commencement. I suggest moving the 4x first, as to non create a negative.
| 8x - 4x - 10 = 4x - 4x + 2 | Subtract 4x from each side |
| 4x - ten = two | Simplify |
Now nosotros are back to a bones two-step equation.
| 4x - 10 + 10 = two + 10 | Add 10 from each side |
| 4x = 12 | Simplify |
| \dfrac{4x}{4} = \dfrac{12}{iv} | Split each side past iv |
| x = 3 | Simplify |
To check you answer, you tin simplify substitute 3 into the variable to see if the equation is true:
| 8x - x = 4x + 2 | Original equation |
| viii(iii) - 10 = 4(3) + 2 | Substitute |
| 24 - ten = 12 + 2 | Simplify |
| 14 = fourteen \checkmark | Respond confirmed |
Thus, x = 3 is the right solution.
Render to the Table of Contents
Below is a short video from Mike DeVor showing more than examples of solving multi-step equations:

Now that we have been introduced to Multi-Step Equations, allow'south get those brain gears in motility and wait at some more than challenging examples!
Multi-step equations with fractions
When dealing with an equation with more than one fraction, the easiest fashion to solve the equation is by finding the Least Common Denominator. The least common denominator is the smallest number that can exist a common denominator for a set of fractions.
Once we find the least common denominator, we will multiply each term by this value to eliminate the fraction. Hither is an example of a multi-step equation with fractions:
Solve for y in the following equation:
\dfrac{5y}{six} - \dfrac{ane}{four} = \dfrac{3y}{4} + \dfrac{i}{two}
The denominators above are 2, 4, half-dozen , therefore the least mutual denominator for these numbers is 12 . So we will multiply each term past 12 .
| 12 \cdot \dfrac{5y}{6} - 12 \cdot \dfrac{1}{4} = 12 \cdot \dfrac{3y}{4} + 12 \cdot \dfrac{1}{2} | Multiply each term by 12 |
| \dfrac{60y}{6} - \dfrac{12}{four} = \dfrac{36y}{four} + \dfrac{12}{2} | Issue of multiplication |
| 10y - three = 9y + half-dozen | Simplify |
| 10y - 9y - iii = 9y - 9y + 6 | Subtract 9y from each side |
| y - 3 = six | Simplify |
| y - 3 + iii = 6 + 3 | Add 3 to each side |
| y = 9 | Simplify |
To check your answer, you can substitute 9 into the variable to see if the equation is true:
| \dfrac{5y}{6} - \dfrac{i}{4} = \dfrac{3y}{four} + \dfrac{ane}{2} | Original equation |
| 10y - three = 9y + 6 | Simplified equation (all terms multiplied past 12 ) |
| 10 \cdot 9 - three = 9 \cdot ix + 6 | Substitute |
| ninety - iii = 81 + vi | Simplify |
| 87 = 87 \checkmark | Reply confirmed |
Therefore, y = 9 is the correct solution.
Return to the Tabular array of Contents
Multi-step equations with distributive holding
Case 1
Solve for z in the following equation:
| 2(3z - iv) = x | Original equation |
| 2(3z) - 2(iv) = 10 | Distributive Property |
| 6z - eight = x | Simplify |
| 6z - eight + viii = ten + 8 | Add 8 to each side |
| 6z = 18 | Simplify |
| \dfrac{6z}{half-dozen} = \dfrac{18}{six} | Divide each side past half-dozen |
| z = 3 | Simplify |
To cheque you answer, you tin substitute iii into the variable to see if the equation is true:
| 2(3z - 4) = 10 | Original equation |
| 2(3 \cdot three - 4) = 10 | Substitute |
| ii(9 - 4) = 10 | Simplify |
| 2(5) = 10 | Simplify |
| ten = 10 \checkmark | Answer confirmed |
Thus, z = iii is the correct solution.
Instance 2
Solve for g in the following equation:
| iii(one thousand + three) - four = 2(m - 2) | Original equation |
| three(m) + 3(three) - 4 = ii(m) - 2(2) | Distributive Property |
| 3m + 9 - 4 = 2m - iv | Simplify |
| 3m + 5 = 2m - four | Combine similar terms |
| 3m - 2m + v = 2m - 2m - 4 | Decrease 2m from each side |
| m + five = - iv | Simplify |
| m + 5 - 5 = - 4 - five | Subtract 5 from each side |
| m = -nine | Simplify |
To cheque you reply, you lot can simplify substitute -9 into the variable to encounter if the equation is truthful:
| 3(m + iii) - 4 = two(thou - ii) | Original equation |
| 3(-nine + three) - 4 = ii(-ix - 2) | Substitute |
| 3(-six) - four = two(-11) | Simplify |
| -18 - 4 = -22 | Combine similar terms |
| -22 = -22 \checkmark | Answer confirmed |
Thus, m = -9 is the correct solution.
Return to the Table of Contents
Multi-step equation word problems
Case ane
Rob owns a java shop and is looking at finding a new java distributor for his beans. Benefactor A sells their beans for \$5 a pound, plus a flat \$10 shipping fee. Distributor B sells their beans for \$two a pound, plus \$1 per pound for aircraft, plus a \$xl processing fee. What corporeality of pounds, p , would exist a breakeven bespeak for the two companies?
Solution
First, allow's create an equation for the state of affairs:
| 5p + 10 = 2p + 1p + 40 | Original equation |
| 5p + 10 = 3p + 40 | Combine like terms |
| 5p -3p + ten = 3p - 3p + 40 | Subtract 3p from each side |
| 2p + 10 = 40 | Simplify |
| 2p + ten - 10 = twoscore - 10 | Decrease ten from each side |
| 2p = 30 | Simplify |
| \dfrac{2p}{2} = \dfrac{30}{ii} | Dissever each side by 2 |
| p = 15 | Simplify |
To check you respond, you can simplify substitute fifteen into the variable to see if the equation is true:
| 5p + 10 = 2p + 1p + 40 | Original equation |
| 5(15) + 10 = ii(xv) + 1(xv) + 40 | Substitute |
| 75 + 10 = 30 + 15 + 40 | Simplify |
| 85 = 85 \checkmark | Respond confirmed |
Therefore, the breakeven point for Benefactor A and Distributor B would be fifteen pounds.
Example 2
Sam goes to a bookstore with a coupon for \$five off a book. The coupon is immune to exist used equally many times every bit Sam wants. He ends up buying 3 books that all cost the same corporeality of money. The full cost for the books was \$45 . How much did each volume cost, c , before the coupon was applied?
Solution
First, let'southward ready an equation that models the state of affairs:
3(c - five) = 45
Since each book costs the same amount, we denote this amount by the variable, c . Then we applied the \$5 coupon to each book, and finally, we will multiply the toll of each book afterwards the coupon by 3 .
Now, simply solve for c like whatever other multi-step equation:
| three(c) - iii(5) = 45 | Distribute the iii |
| 3c - 15 = 45 | Simplify |
| 3c - fifteen + 15 = 45 + xv | Add fifteen to both sides |
| 3c = threescore | Simplify |
| \dfrac{3c}{3} = \dfrac{60}{three} | Split both sides by three |
| c = 20 | Solved |
Therefore, each book cost \$20 earlier the coupon was applied.

Keys to Retrieve: Solving Multi-Step Equations
- A multi-step equation is an equation that requires two or more steps to solve.
- When solving: think whatever y'all do to ane side, y'all must practice to the other.
- To solve multi-step equations with fractions, you tin can multiply each term by the least common denominator to eliminate the fractions outset.
- To bank check the solution, but substitute the value into the variable to see if the equation is true.
- Y'all can model existent-life situations with an equation and solve for a correct solution.
Return to the Tabular array of Contents
Read these other helpful posts:
- Solving 1-Step Equations
- Solving Ii-Step Equations
- Forms of Linear Equations
- View ALL Algebra 1 Review Guides
Return to the Table of Contents
Solving Multi Step Equations Answers,
Source: https://www.albert.io/blog/solving-multi-step-equations/
Posted by: ferrarifichalfic.blogspot.com


0 Response to "Solving Multi Step Equations Answers"
Post a Comment